

(1984) suggested that it is rather the relationships among the lightness percepts, instead of luminance or reflectance, that determine perceived transparency, which Metelli later adopted ( Metelli, 1985). Metelli determined a relationship between the reflectances of the fields of the two disks that predicts the amount of perceived transparency.

When the sliced disk is spun fast enough, optical mixing of the reflected light leads to the appearance of a solid, non rotating transparent filter in front of the bipartite field. The sliced rotating disk was painted with one uniform gray value. In some of his experiments, the stationary disk was painted such that the left half had one gray value and the right half had another, creating a vertical split in luminance down the middle (a bipartite field) that the sliced rotating disk overlaid. The episcotister is a device that Metelli used that rotates a sliced disk in front of a complete and stationary disk.

However, it was Metelli’s work (1970, 1974) that has had a more lasting impact on the study of perceived transparency, resulting in the classical Metelli or episcotister model of transparency, whose original equation is coincidentally used in computer graphics in a form of alpha blending known as Porter and Duff’s “over” operator ( Porter & Duff, 1984 Foley et al., 1990), although the “over” operator actually models the effect of an opaque surface with partial coverage, whose optical effects are physically different from the optical effects of transparent objects ( McGuire, 2019). Helmholtz (1867) and Koffka (1935) wrote about the perception of transparency in their books, where Helmholtz described the percept of transparency as “a transparent colored veil … spread over the field” and Koffka referred to it as “color scission,” in which the visual system can assign more than one color to the same image region, effectively splitting that region into layers and perceiving a colored filter overlaying another colored object. The conditions that lead to the perception of flat transparent filters and the factors that determine the perceived color of a flat filter have been extensively studied. Transparent objects allow some light to pass through their bodies, as opposed to opaque objects, which only absorb and reflect light. The RSD seems instead to be related to the perceived “clarity” of glass objects. We conclude that the RMC is probably the strongest factor for determining the color. Considering that, the RMC and robust ratio models generalized more than the RSD, with the RMC performing best across the stimuli we tested. The original convergence model does not fully generalize but does not perform poorly, and with modifications, we find that curved transparent objects cause a convergence of filtered colors toward a point in color space, similar to flat filters. We applied four models from flat filter studies: the convergence model, the ratios of either the means (RMC) or standard deviations (RSD) of cone excitations, and a robust ratio model.
#20 examples of transparent objects Patch
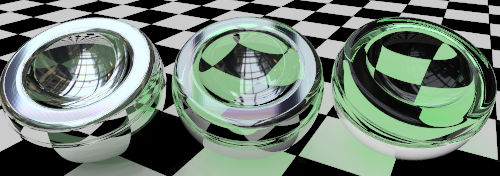
Observers’ matches differed from the mean, the most frequent, and the most saturated color of the transparent stimuli, whereas the brightest regions captured the chromaticity, but not the lightness, of patch matches. Two instructions were tested: simply match the color of the glass object and the test element (patch and flat filter) or match the color of the dye that was used to tint the transparent object (patch). Here, we investigate color-matching experiments with curved transparent objects for different matching stimuli: a uniform patch and a flat filter. While they have provided valuable insight, these studies have not captured all aspects of transparency, like caustics, specular reflections/highlights, and shadows. Studies on colored transparent objects have elucidated potential mechanisms, but these studies have mainly focused on flat filters overlaying flat backgrounds.


 0 kommentar(er)
0 kommentar(er)
